The behavior of fluids in thin tubes and narrow crevices - simulation in the world of microfluidics and capillaries.
Introduction
Capillary action is the physical process by which liquids can flow along narrow tubes or capillaries, even against gravity, due to surface tension.
Capillary action occurs when the adhesive forces between a liquid and the surface of a solid tube are stronger than the cohesive forces within the liquid itself. The narrower the diameter of the capillary, the higher a liquid can rise in a capillary.
Examples of capillary flow can be found in nature, where water is transported upward from the roots of plants through fine capillaries in the stems and leaves. But also the supply of the body with blood and oxygen would be unthinkable without the fine veins, which are not called capillaries for nothing.
Capillary flow has practical applications in engineering, such as microfluidics, where it is used to control, manipulate and transport fluids in tiny tubes or channels. Applications exist from inkjet printers to medical diagnostic equipment.
Sometimes the capillary action is also undesirable. In the construction industry, for example, it can cause water to penetrate concrete or masonry and cause damage. In medicine, it can cause fluid to penetrate tissue unintentionally. Capillary action can also be a problem in microelectronics when liquids penetrate the tiny gaps between chips and cause short circuits.
In contrast, it plays a minor role when drinking with a straw. Here, the usual diameter is 6 mm. Assuming water, the rise is only 4.64 mm, not enough for the cocktail to flow into the mouth on its own. A thin tube is also of little help, because otherwise it takes too long to drink your cocktail.
Analytical calculation
The rising height of a liquid in a capillary can be calculated analytically using the so-called Jurin equation. This equation describes the relationship between the rising height of a liquid in a capillary and the surface tension and diameter of the capillary.
The Jurin equation is:
h = (2 * γ * cos(θ)) / (ρ * g * r)
Where:
h for the rising height of the liquid in the capillary
γ for the surface tension of the liquid
θ for the contact angle between the liquid and the capillary wall
ρ for the density of the liquid
g for the acceleration due to gravity
r for the radius of the capillary

Thus, to calculate the rise height h, the values for γ, θ, ρ, g and r must be known. The surface tension γ can be determined by a surface tension meter. The contact angle θ depends on the type of capillary wall and the liquid and gaseous medium and can also be determined experimentally. The density ρ of the liquid can be determined from tables or by measurements, and the acceleration due to gravity of g = 9.81 m/s2 has been known since 1686, if I remember correctly
The calculation of the rise height, but also of the rise velocity due to capillary action, is of great importance in many fields, such as biology, medicine, chemistry and materials science. Knowledge of the height of rise allows processes to be optimized and materials to be targeted with specific properties.
So how good is simulation at calculating capillary action?
For this purpose, a benchmark is described below, which we at Merkle CAE Solutions have recalculated on a simple model with Star CCM+ and compared with the analytical result.
Our model consists of a segment of a glass tube with a radius of 0.25 mm.

The characteristic values for water versus air required in the Jurin equation, as well as for the simulation model in Star CCM+, are:
The surface tension water / air γ= 0.0728 N/m at 20 °C.
The contact angle θ water / air is about θ = 20° or 0.35 rad.
The density ρ of water is about 1000 kg/m3 at 20 °C.
The acceleration due to gravity g is about 9.81 m/s2.
The radius r = 0.25 mm of the capillary
With these values, the rise height of water in a capillary can be calculated.
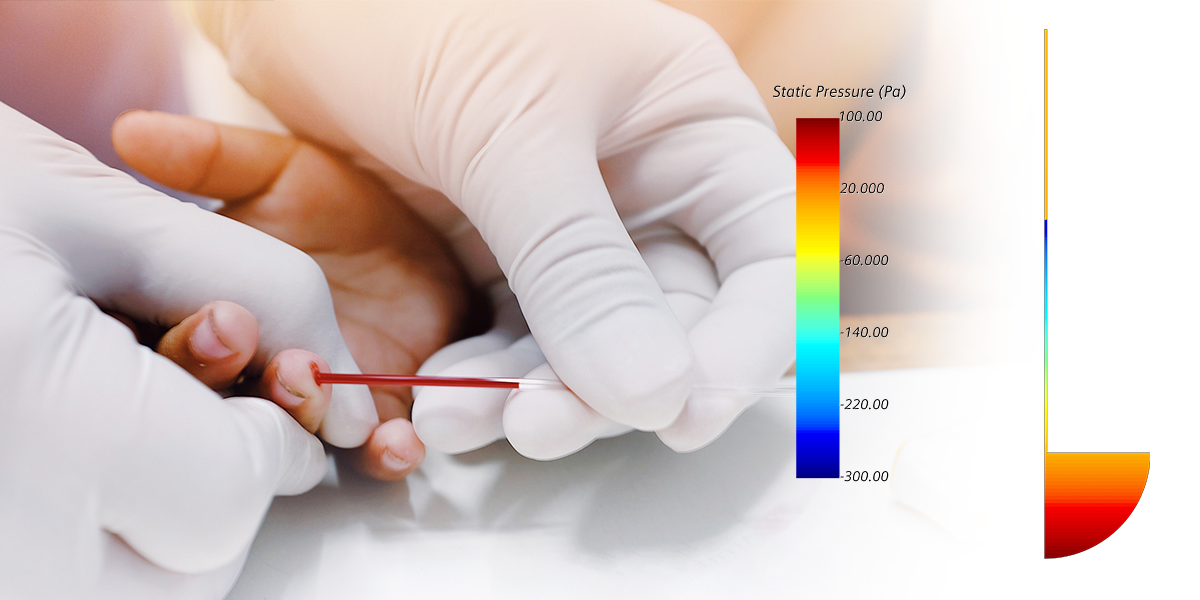
The Jurinformel gives a value of 56 mm here, the CFD calculation at Merkle CAE Solutions shows a value of 52.5 mm after 2 seconds. The deviation is about 6%. However, the value could further approach the steady-state value with longer real times in the calculation (see Figure 2).
Figure 3 shows the plausible surface, the so-called meniscus. Figure 4 shows the pressure distribution in the tube.
If one takes a higher contact angle of 60°, the results become almost congruent (about 28 mm). No wonder why tutorials prefer to work with the higher values.

But before a discussion about the accuracy breaks out here: It is recommended in any case to adjust the calculation model with simple tests exactly for the respective case. The influence of the contact angle in particular is very large.
There are also contradictory statements on the Internet about the Jurin equation, for example, the radius r is included once and the diameter d once for the same type of formula. The formula given here with r should be correct.
The advantage of CFD simulation with Merkle CAE Solutions is that the effects can be mapped even with very complex geometry, where the Jurin equation stretches the wings. In addition, temporal effects can also be taken into account, which play an essential role, especially in the case of faster cycle times of laboratory measuring equipment.

Yours Stefan Merkle

PS: During the research, Chat GTP wanted me to believe that insects drink with their feet due to the capillary effect. But then I could not quite believe that. Maybe the knowledge before 2022 is outdated here and there are new findings... (which I also do not believe). Probably Chat GTP adapts to the general political weather situation, after all, our politics has nothing to do with physics for a long time.
PPS: Mercury has a very high surface tension of 484 mN /m and a contact angle of between 135-142 ° compared to most solids. And indeed, it results in a negative rise of -25.9 mm for our drinking straw. So the mirror in the tube is below the surface. Faintly, I remember a physics experiment in school that showed exactly that. Only the question remains, who wants to drink mercury with the tube and why.
PPPS: Maik only has experience with 40% alcohol-water mixtures. Here the contact angle is almost 0, the surface tension is 22.55 mN/m and the density is 830 kg/m³. The rise height is then only 1.84 mm and, due to the alcohol consumption, is then no longer perceived by him.